Formula of eccentricity of hyperbola

The eccentricity of hyperbola is greater than 1. The eccentricity of hyperbola helps us to understand how closely in circular shape, it is related to a circle.

Eccentricity in a conic section is a unique character of its shape and is a value that does not take negative real numbers. Generally, eccentricity gives a measure of how much a shape is deviated from its circular shape. We already know that the four basic shapes that are formed on intersection of a plane with a double-napped cone are: circle, ellipse, parabola , and hyperbola. The characteristics of these shapes are determined by the value of eccentricity. In the maths article, we shall learn about eccentricity and its values for different conic sections. We shall also individually learn about the eccentricities of circle, ellipse, hyperbola, as well as parabola and the ways to find it using solved examples for better understanding of the concept.

Formula of eccentricity of hyperbola

The eccentricity in the conic section uniquely characterises the shape where it should possess a non-negative real number. In general, eccentricity means a measure of how much the deviation of the curve has occurred from the circularity of the given shape. We know that the section obtained after the intersection of a plane with the cone is called the conic section. We will get different kinds of conic sections depending on the position of the intersection of the plane with respect to the plane and the angle made by the vertical axis of the cone. In this article, we are going to discuss the eccentric meaning in geometry, and eccentricity formula and the eccentricity of different conic sections such as parabola, ellipse and hyperbola in detail with solved examples. The eccentric meaning in geometry represents the distance from any point on the conic section to the focus divided by the perpendicular distance from that point to the nearest directrix. Generally, the eccentricity helps to determine the curvature of the shape. If the curvature decreases, the eccentricity increases. Similarly, if the curvature increases, the eccentricity decreases. We know that there are different conics such as a parabola, ellipse, hyperbola and circle. The eccentricity of the conic section is defined as the distance from any point to its focus, divided by the perpendicular distance from that point to its nearest directrix. The eccentricity value is constant for any conics.

Learn about Parabola Ellipse and Hyperbola. Area segment Circle.

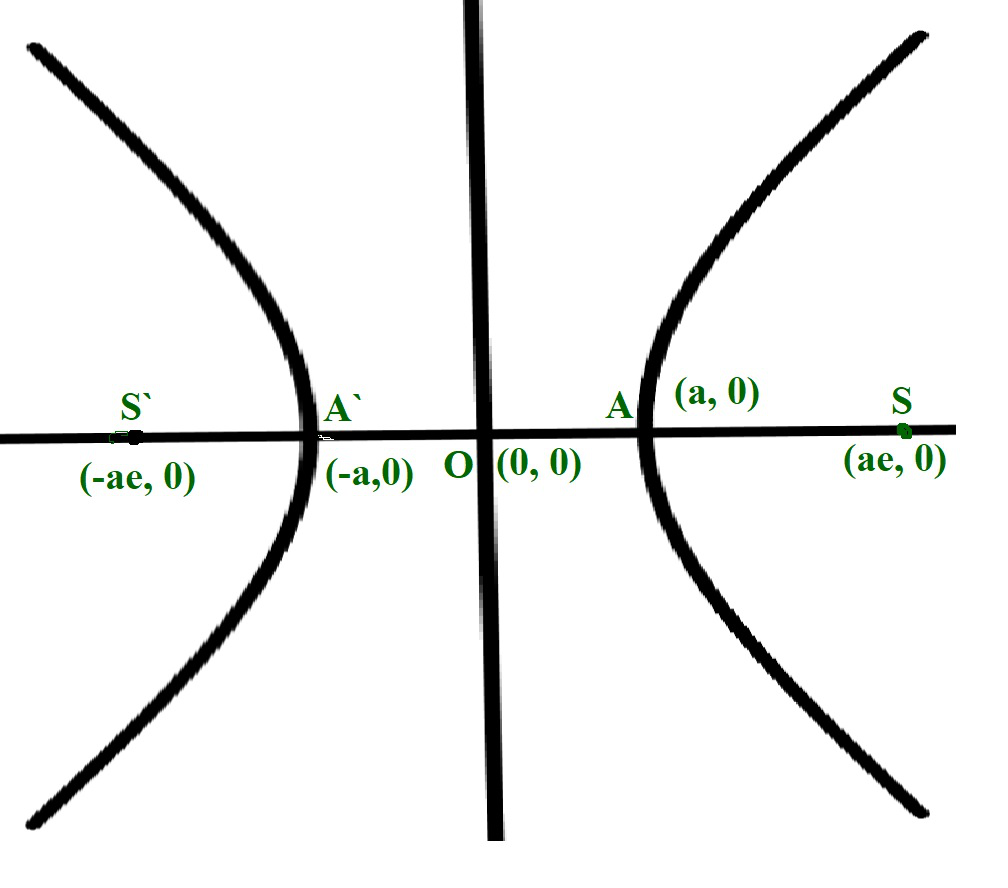
A hyperbola is the set of all points in a plane, the difference of whose distances from two fixed points in the plane is constant. The two fixed points are the foci and the mid-point of the line segment joining the foci is the center of the hyperbola. The line through the foci is called the transverse axis. Also, the line through the center and perpendicular to the transverse axis is called the conjugate axis. The points at which the hyperbola intersects the transverse axis are called the vertices of the hyperbola. We take a point P at A and B as shown above. Therefore, by the definition of a hyperbola, we have.

The eccentricity of any curved shape characterizes its shape, regardless of its size. The four curves that get formed when a plane intersects with the double-napped cone are circle, ellipse, parabola, and hyperbola. Their features are categorized based on their shapes that are determined by an interesting factor called eccentricity. The circles have zero eccentricity and the parabolas have unit eccentricity. The ellipses and hyperbolas have varying eccentricities. Let us learn more in detail about calculating the eccentricities of the conic sections.

Formula of eccentricity of hyperbola

In mathematics, a hyperbola is an important conic section formed by the intersection of the double cone by a plane surface, but not necessarily at the center. A hyperbola is symmetric along the conjugate axis, and shares many similarities with the ellipse. Concepts like foci, directrix, latus rectum, eccentricity, apply to a hyperbola. A few common examples of hyperbola include the path followed by the tip of the shadow of a sundial, the scattering trajectory of sub-atomic particles, etc. Here we shall aim at understanding the definition, formula of a hyperbola, derivation of the formula, and standard forms of hyperbola using the solved examples. A hyperbola, a type of smooth curve lying in a plane, has two pieces, called connected components or branches, that are mirror images of each other and resemble two infinite bows. A hyperbola is a set of points whose difference of distances from two foci is a constant value. This difference is taken from the distance from the farther focus and then the distance from the nearer focus.

Mass effect 3 blue suns quest

In other words, the distance from the fixed point in a plane bears a constant ratio less than the distance from the fixed-line in a plane. Your email address will not be published. The projectile covers the longest horizontal distance when it is thrown at 45 degrees. The line through O perpendicular to the x-axis be the y-axis. Learn Eccentricity Of Hyperbola with tutors mapped to your child's learning needs. For an Ellipse, the value of Eccentricity is equal to. Stay tuned to the Testbook App for more updates on related topics from Mathematics, and various such subjects. Join courses with the best schedule and enjoy fun and interactive classes. At Eccentricity equal to 0. Commercial Maths. Hyperbolic Function Foumlas. Get ready for all-new Live Classes!

A hyperbola is a two-dimensional curve in a plane. It takes the form of two branches that are mirror images of one another that together form a shape similar to a bow.

The two important terms to refer to before we talk about eccentricity is the focus and the directrix of the hyperbola. Learn Eccentricity Of Hyperbola with tutors mapped to your child's learning needs. We obtain a line. In other words, the distance from the fixed point in a plane bears a constant ratio equal to the distance from the fixed-line in a plane. For a Parabola, the value of Eccentricity is 1. Area Of A Circle Formula. Already booked a tutor? Eccentricity for the hyperbola is? What is the eccentricity of a circle? Derivation of Eccentricity of Hyperbola 4. So, we can say that the value of eccentricity for any circle is always zero. Explore math program.