Formula of inscribed angle

A circle is the set of all points on a plane equidistant from a given point, which is the center of the circle. The only way to gather all the points that are the same distance from a point is to create a curved line. A circle has other parts, too, formula of inscribed angle, not important to this discussion: secant and point of tangency are two such parts. Circles are almost always indicated by the mathematical symbol followed by the circle's letter designation, its center point.

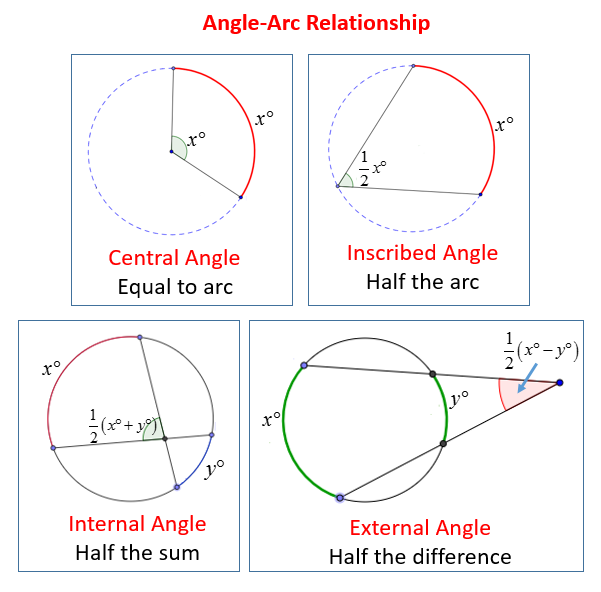
In geometry , an inscribed angle is the angle formed in the interior of a circle when two chords intersect on the circle. It can also be defined as the angle subtended at a point on the circle by two given points on the circle. The inscribed angle theorem relates the measure of an inscribed angle to that of the central angle subtending the same arc. The inscribed angle theorem appears as Proposition 20 on Book 3 of Euclid's Elements. Therefore, the angle does not change as its vertex is moved to different positions on the circle.

Formula of inscribed angle

Definition: An inscribed angle is an angle whose vertex lies on the circumference of the circle. The vertex is the common endpoint of the two sides of the angle. An inscribed angle can be defined as the angle subtended at a point on the circle by two given points on the circle. An inscribed angle is an angle formed in the interior of a circle by two chords that have a common endpoint on the circle. The inscribed angle is used in many proofs of elementary Euclidean geometry of the plane. Inscribed angle is the basis for several other theorems related to the power of a point with respect to a circle. Inscribed angle is a very important part of the circle theorems. Four circle theorems are directly based on the inscribed angle. Angle at the center is double the angle at the circumference. An angle inscribed in a semi-circle is a right angle. In a cyclic quadrilateral, there are four inscribed angles.

A common figure involving a circle is an inscribed angle. Now draw line OV and extend it past point O so that it intersects the circle at point E.

A circle is unique because it does not have any corners or angles, which makes it different from other figures such as triangles , rectangles, and triangles. But specific properties can be explored in detail by introducing angles inside a circle. For instance, the simplest way to create an angle inside a circle is by drawing two chords such that they start at the same point. This might seem unnecessary at first, but by doing so, we can employ many rules of trigonometry and geometry , thus exploring circle properties in more detail. Explore our app and discover over 50 million learning materials for free.

Home » Geometry » Angle » Inscribed Angle. An inscribed angle is an angle whose vertex lies on the circumference of a circle while its two sides are chords of the same circle. The arc formed by the inscribed angle is called the intercepted arc. In order to prove this theorem, we need to consider three separate cases. Each of them will differ based on where the center lies in comparison to the inscribed angle. Case 1: Prove Inscribed Angle Theorem when the inscribed angle is between a chord and the diameter of a circle. Case 2: Prove Inscribed Angle Theorem when the diameter is between the two chords forming the inscribed circle. Case 3: Prove Inscribed Angle Theorem when the diameter is outside the two chords forming the inscribed angle. Inscribed angle of a circle can be determined if its corresponding central angle is known by using the formula derived from the inscribed angle theorem given below:. Solve the missing angle x in the diagram given below.

Formula of inscribed angle

The inscribed angle theorem mentions that the angle inscribed inside a circle is always half the measure of the central angle or the intercepted arc that shares the endpoints of the inscribed angle's sides. In a circle, the angle formed by two chords with the common endpoints of a circle is called an inscribed angle and the common endpoint is considered as the vertex of the angle. In this section, we will learn about the inscribed angle theorem, the proof of the theorem, and solve a few examples. The inscribed angle theorem is also called the angle at the center theorem as the inscribed angle is half of the central angle. Since the endpoints are fixed, the central angle is always the same no matter where it is on the same arc between the endpoints.

Sony vaio laptop

Enter the central angle , and our calculator will find the inscribed angle for you. Find tutors nearby. With circles, geometry becomes at once more interesting and more difficult. Inscribed quadrilateral Example, StudySmarter Originals. Out of these, the cookies that are categorized as necessary are stored on your browser as they are essential for the working of basic functionalities of the website. Taking a short quiz. Fact-checked by Paul Mazzola. In a circle, inscribed angles that intercept the same arc are congruent. Choose two points on the circle, and call them V and A. In Elements Angle bisector theorem Exterior angle theorem Euclidean algorithm Euclid's theorem Geometric mean theorem Greek geometric algebra Hinge theorem Inscribed angle theorem Intercept theorem Intersecting chords theorem Intersecting secants theorem Law of cosines Pons asinorum Pythagorean theorem Tangent-secant theorem Thales's theorem Theorem of the gnomon. See also Tangent lines to circles. Lines OV and OA are both radii of the circle, so they have equal lengths. That, of course, is the Inscribed Angle Theorem. Geometry Tutors Charlotte. Inscribed angle theorem - Inscribed angle formula Calculating central angle, inscribed angle, and arc length Using this inscribed angle calculator FAQ.

An inscribed angle is an angle whose vertex is on a circle and whose sides contain chords of a circle. This is different than the central angle, whose vertex is at the center of a circle. If you recall, the measure of the central angle is congruent to the measure of the minor arc.

Free math cheat sheet! If a quadrilateral is inscribed in a circle, which means that the quadrilateral is formed in a circle by chords, then its opposite angles are supplementary. Sign up to highlight and take notes. Using the inscribed angle theorem, we know that the central angle is twice the inscribed angle that intercepts the same arc. Congruent angles have the same degree measure. Angle Formed by Two Intersecting Chords. Inscribed Angle An inscribed angle is an angle with its vertex "on" the circle, formed by two intersecting chords. Inscribed angle theorem - Inscribed angle formula The inscribed angle theorem establishes a relationship between the central and inscribed angles. Head to our arc length calculator! Geometry Tutors Houston. The only way to gather all the points that are the same distance from a point is to create a curved line. Flashcards in Inscribed Angles 2 Start learning. Join over 22 million students in learning with our StudySmarter App. The opposite angles in a cyclic quadrilateral are supplementary.