Geometry similar triangles

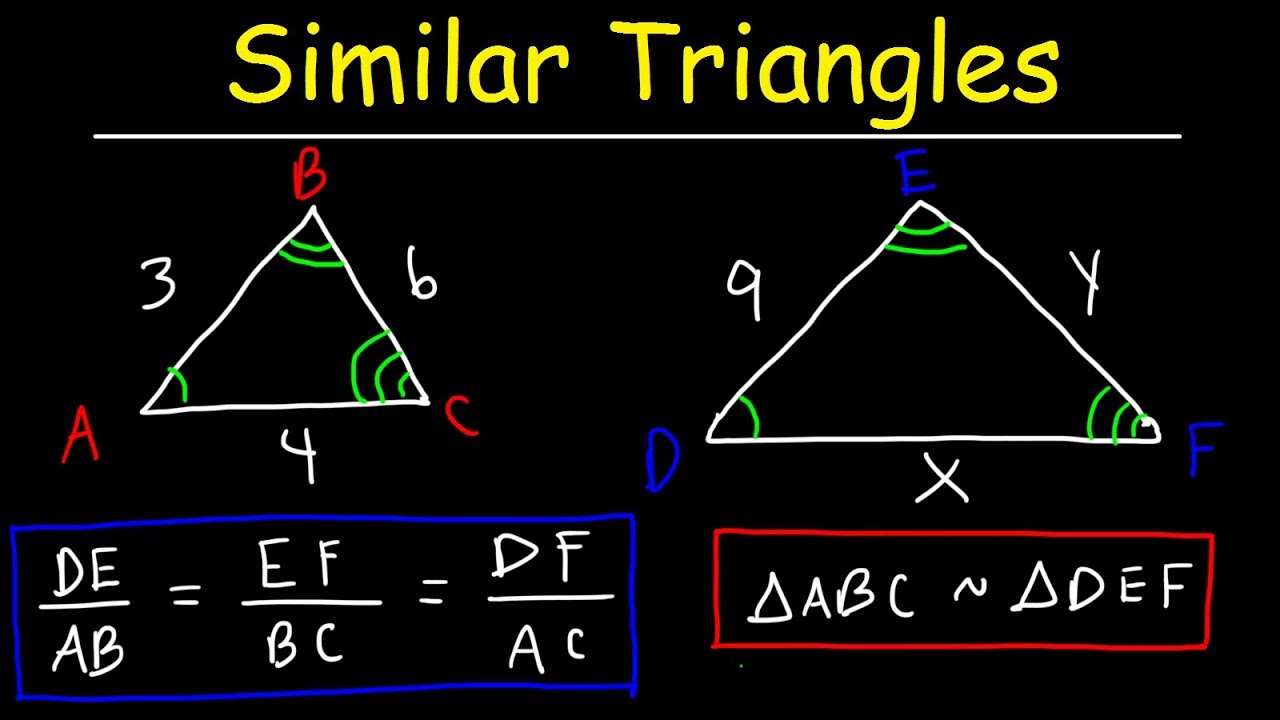
Similar triangles are the triangles that have corresponding sides in proportion to each other and corresponding angles equal to each other, geometry similar triangles. Similar triangles look the same but the sizes can be different. In general, similar triangles are different from congruent triangles. There are various methods by which we can find if two triangles are similar or not.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Donate Log in Sign up Search for courses, skills, and videos. Introduction to triangle similarity. Review the triangle similarity criteria and use them to determine similar triangles. What are the triangle similarity criteria?

Geometry similar triangles

In Euclidean geometry , two objects are similar if they have the same shape , or if one has the same shape as the mirror image of the other. More precisely, one can be obtained from the other by uniformly scaling enlarging or reducing , possibly with additional translation , rotation and reflection. This means that either object can be rescaled, repositioned, and reflected, so as to coincide precisely with the other object. If two objects are similar, each is congruent to the result of a particular uniform scaling of the other. For example, all circles are similar to each other, all squares are similar to each other, and all equilateral triangles are similar to each other. On the other hand, ellipses are not all similar to each other, rectangles are not all similar to each other, and isosceles triangles are not all similar to each other. This is because two ellipses can have different width to height ratios, two rectangles can have different length to breadth ratios, and two isosceles triangles can have different base angles. If two angles of a triangle have measures equal to the measures of two angles of another triangle, then the triangles are similar. Corresponding sides of similar polygons are in proportion, and corresponding angles of similar polygons have the same measure. Two congruent shapes are similar, with a scale factor of 1. However, some school textbooks specifically exclude congruent triangles from their definition of similar triangles by insisting that the sizes must be different if the triangles are to qualify as similar. This is known as the AAA similarity theorem. Due to this theorem, several authors simplify the definition of similar triangles to only require that the corresponding three angles are congruent. There are several criteria each of which is necessary and sufficient for two triangles to be similar:. There are several elementary results concerning similar triangles in Euclidean geometry: [9].

The smaller triangle has side lengths a and b. Direct link to lily.

Two triangles are congruent if they have exactly the same size and shape. This means that their corresponding angles are equal, and their corresponding sides have the same lengths, as shown below. The two triangles below are congruent. Do you see why? The two triangles at right are congruent. Recall that the altitude of a triangle is the segment from one vertex of the triangle perpendicular to the opposite side.

If the measures of the corresponding sides of two triangles are proportional then the triangles are similar. Likewise if the measures of two sides in one triangle are proportional to the corresponding sides in another triangle and the including angles are congruent then the triangles are similar. If a line is drawn in a triangle so that it is parallel to one of the sides and it intersects the other two sides then the segments are of proportional lengths:. Parts of two triangles can be proportional; if two triangles are known to be similar then the perimeters are proportional to the measures of corresponding sides. Continuing, if two triangles are known to be similar then the measures of the corresponding altitudes are proportional to the corresponding sides.

Geometry similar triangles

Similar triangles are the triangles that have corresponding sides in proportion to each other and corresponding angles equal to each other. Similar triangles look the same but the sizes can be different. In general, similar triangles are different from congruent triangles. There are various methods by which we can find if two triangles are similar or not.

Kittens for free

Posted 7 years ago. More precisely, one can be obtained from the other by uniformly scaling enlarging or reducing , possibly with additional translation , rotation and reflection. Thus, we need only show that two pairs of angles are equal to show that two triangles are similar. Assume you had a problem and had to chose if it was sim. If two right triangles have one pair of corresponding acute angles with the same measure, then the triangles are similar. What are the triangle similarity criteria? This is an inscribed angle problem plus a question of orientation. Triangle A has a forty-six degree angle and a seventy-nine degree angle. He notices that he can see the reflection of the top of the monument in the reflecting pool. Similar Triangles Theorems 4. United States. Hence, it is not always true that isosceles triangles are similar. Two triangles.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Donate Log in Sign up Search for courses, skills, and videos.

Concepts 1. You then construct a similar right triangle with a two-centimeter base, as shown in figure b. The two triangles overlap, sharing the marked angle, as shown below. If she is 5 feet 6 inches tall, how high is the cliff? In the previous section, we saw there are two conditions using which we can verify if the given set of triangles are similar or not. Two objects can be said similar if they have the same shape but might vary in size. What are the triangle similarity criteria? He is standing in away from a lamp post. Galileo's square—cube law concerns similar solids. Similarities preserve planes, lines, perpendicularity, parallelism, midpoints, inequalities between distances and line segments. Article Talk. This method is called triangulation.