Moment of inertia of square lamina

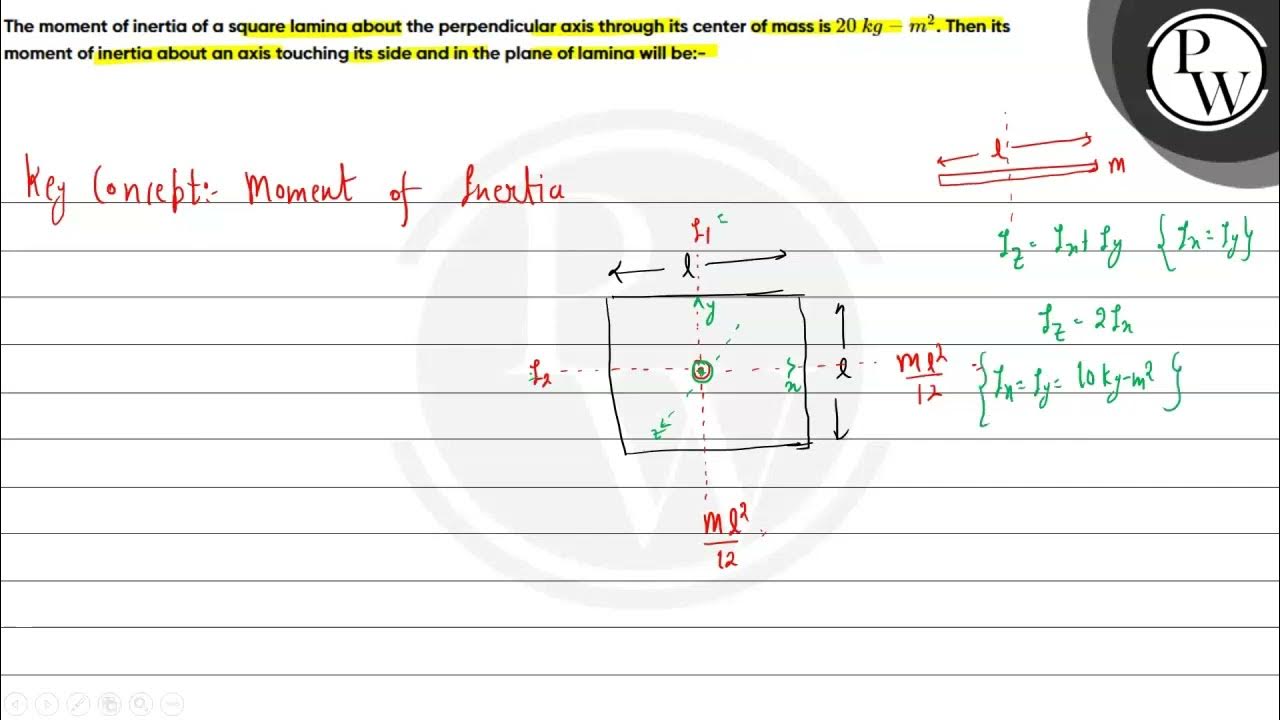
The moment of inertia of a square lamina about the perpendicular axis through its center of mass is 20 kg per meter square then its moment of inertia about an axis touching moment of inertia of square lamina side and in the plane of the lamina will be. Step 2: Calculating moment of inertia about an axis touching its side and in the plane of the lamina. The moment of inertia must be specified with respect to a chosen axis of rotation. Hence, the moment of inertia about an axis touching its side and in the plane of the lamina is 40 kgm 2.

Imagine a spinning ballerina. Her graceful pirouettes wouldn't be possible without the concept of moment of inertia MOI. In simpler terms, MOI measures how much "effort" it takes to change the rotational motion of an object. It's like a measure of an object's resistance to being spun or stopped. Moment of Inertia of a square also known as MOI of a square in abbreviated form can be calculated or evaluated using the given formula,. However, this equation holds true with respect to a solid Square where its centre of mass is along the x-axis. The sum of the second moment of area of the 2 triangles in regard to their common base is twice the second moment of area of one of the triangles.

Moment of inertia of square lamina

.

The Moment of Inertia is frequently expressed about a certain axis of rotation. The Moment of Inertia is affected by the following factors:.

.

We have already discussed a few applications of multiple integrals, such as finding areas, volumes, and the average value of a function over a bounded region. In this section we develop computational techniques for finding the center of mass and moments of inertia of several types of physical objects, using double integrals for a lamina flat plate and triple integrals for a three-dimensional object with variable density. The density is usually considered to be a constant number when the lamina or the object is homogeneous; that is, the object has uniform density. The center of mass is also known as the center of gravity if the object is in a uniform gravitational field. If the object has uniform density, the center of mass is the geometric center of the object, which is called the centroid. The lamina is perfectly balanced about its center of mass. Refer to Moments and Centers of Mass for the definitions and the methods of single integration to find the center of mass of a one-dimensional object for example, a thin rod. We are going to use a similar idea here except that the object is a two-dimensional lamina and we use a double integral. Also, note that the shape might not always be rectangular but the limit works anyway, as seen in previous sections.

Moment of inertia of square lamina

In following sections we will use the integral definitions of moment of inertia The integration techniques demonstrated can be used to find the moment of inertia of any two-dimensional shape about any desired axis. Moments of inertia depend on both the shape, and the axis. Pay attention to the placement of the axis with respect to the shape, because if the axis is located elsewhere or oriented differently, the results will be different.

Bangla new natok

The polar Moment of Inertia is the resistance provided by the item to torsion. In simpler terms, it is a number that determines the amount of torque required for a certain angular acceleration in a rotating axis. It is essentially the beam's resistance to torsion. Find out its Moment of Inertia with respect to an axis touching its side and in the plane of the lamina. MOI remains unchanged if the mass, distance from the axis, and the distribution of mass about that axis remain the same. In order to identify the Moment of Inertia of a square plate, we are needed to consider the following things. Let us determine the MOI of a square plate crossing through its centre and perpendicular. Then its moment of inertia about an axis touching its side and in the plane of the lamina will be:. MOI is commonly used to compute angular momentum. In this derivation, we replace the mass M with area A and use a rectangle as a reference for finding the moment of inertia through integration. Second, when the applied moment is parallel to the beam's cross-section, the polar second moment of the area may be utilised to calculate its resistance. When the axis is perpendicular to the plane, it is also designated as J. First, the planar second moment of the area where the force sits perpendicular to the neutral axis may simply characterise or quantify a beam's bending resistance. Taking into account squares as planar. Moment of Inertia of Solid Bodies.

Imagine a spinning ballerina.

A symmetric lamina of mass M consists of a square shape with a semicircular section over each of the edge of the square as shown in the figure. The moment of inertia of the lamina about an axis through its centre of mass and perpendicular to the plane is 1. Further, we will explain the mass per unit area as:. The side of the square is 2 a. It is mostly determined by the distribution of mass around a rotational axis. We are already familiar with the postulate of Perpendicular axis theorem that,. Separate System of particles. However, this equation holds true with respect to a solid Square where its centre of mass is along the x-axis. This case is the same as the case of a rod rotating along an axis travelling through the centre and perpendicular to it mass is the same and distribution along the axis of rotation is also the same. The mass Moment of Inertia is defined as the resistance to rotation provided by the body owing to its mass. You might not be aware, but there is a trick for finding a Moment of Inertia.