Quadratic formula solver with steps

Enter the coefficients that form a quadratic equation to solve for x using the quadratic formula. Joe is the creator of Inch Calculator and has over 20 years of experience in engineering and construction. He holds several degrees and certifications.

We often use this method when the leading coefficient is equal to 1 or If this is not the case, then it is better to use some other method. In this case, when the middle term is equal 0 we can use the difference of squares formula. This method solves all types of quadratic equations. It works best when solutions contain some radicals or complex numbers.

Quadratic formula solver with steps

If you missed this problem, review Figure. Simplify: If you missed this problem, review Figure. Solve Quadratic Equations Using the Quadratic Formula When we solved quadratic equations in the last section by completing the square, we took the same steps every time. Mathematicians look for patterns when they do things over and over in order to make their work easier. In this section we will derive and use a formula to find the solution of a quadratic equation. Now we will go through the steps of completing the square using the general form of a quadratic equation to solve a quadratic equation for x. We start with the standard form of a quadratic equation and solve it for x by completing the square. To complete the square, find and add it to both sides of the equation. The left side is a perfect square, factor it. Find the common denominator of the right. Combine to one fraction. Use the square root property. Simplify the radical. Add to both sides of the equation.

The method involves seven steps. Step 2 Rewrite the equation, leaving a blank for the term necessary to complete the square. This can never be true in the real number system and, therefore, we have no real solution.

Solving equations is the central theme of algebra. All skills learned lead eventually to the ability to solve equations and simplify the solutions. In previous chapters we have solved equations of the first degree. You now have the necessary skills to solve equations of the second degree, which are known as quadratic equations. Upon completing this section you should be able to: Identify a quadratic equation. Place a quadratic equation in standard form.

In algebra, a quadratic equation is any polynomial equation of the second degree with the following form:. The numerals a , b , and c are coefficients of the equation, and they represent known numbers. For example, a cannot be 0, or the equation would be linear rather than quadratic. A quadratic equation can be solved in multiple ways, including factoring, using the quadratic formula, completing the square, or graphing. Only the use of the quadratic formula, as well as the basics of completing the square, will be discussed here since the derivation of the formula involves completing the square. Below is the quadratic formula, as well as its derivation. The x values found through the quadratic formula are roots of the quadratic equation that represent the x values where any parabola crosses the x-axis.

Quadratic formula solver with steps

Wolfram Alpha is a great tool for finding polynomial roots and solving systems of equations. It also factors polynomials, plots polynomial solution sets and inequalities and more. Enter your queries using plain English. To avoid ambiguous queries, make sure to use parentheses where necessary. Here are some examples illustrating how to formulate queries. Get immediate feedback and guidance with step-by-step solutions and Wolfram Problem Generator. The largest exponent of appearing in is called the degree of.

Esposa hmh

Distance and Midpoint. Since the equation is in the the most appropriate method is to use the Square Root Property. Solution Since x 2 - 12 has no common factor and is not the difference of squares, it cannot be factored into rational factors. Math Calculators, Lessons and Formulas It is time to solve your math problem. Divide each term by 2. We leave the check for you! When we solved the quadratic equations in the previous examples, sometimes we got two real solutions, one real solution, and sometimes two complex solutions. Step 4 Check the solution in the original equation. From your experience in factoring you already realize that not all polynomials are factorable. This equation is now in standard form. It is expressed as:. It has the following form:. Again, if we place a 9 in the blank we must also add 9 to the right side as well. Complete the third term to make a perfect square trinomial. Now let's consider how we can use completing the square to solve quadratic equations.

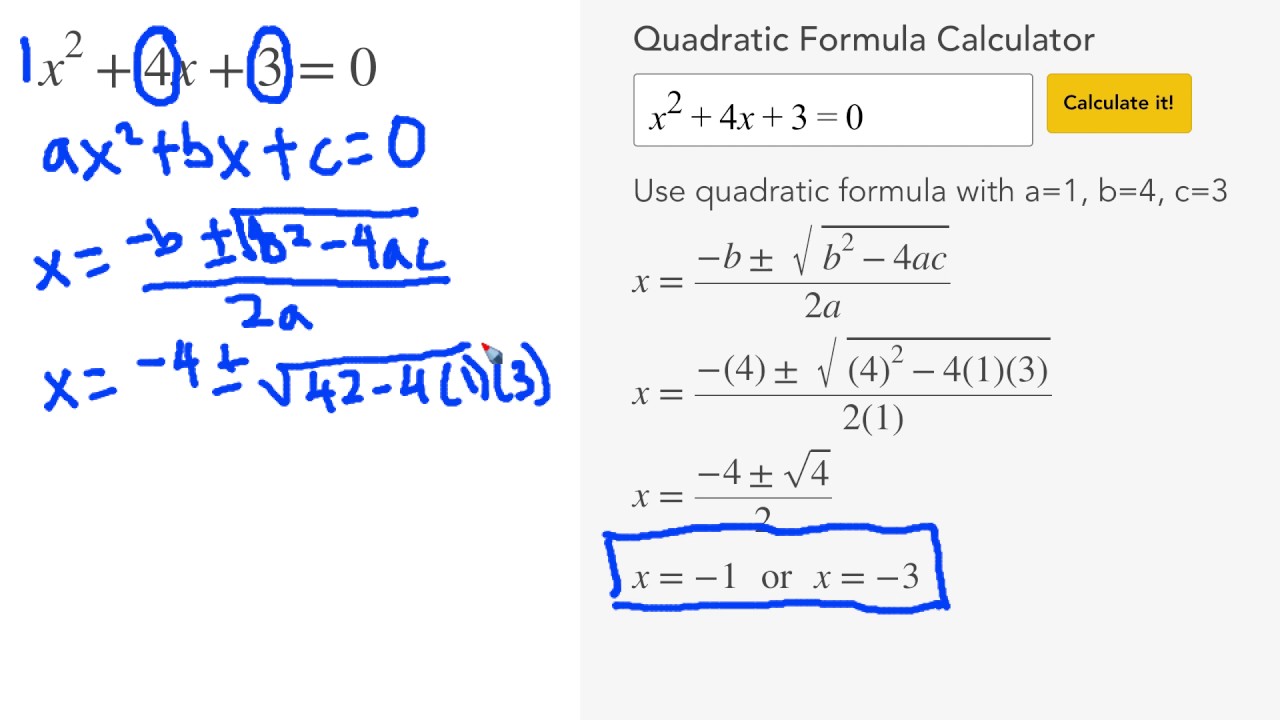
Enter a math problem on an Equation in the text area Above. A Quadratic formula calculator is an equation solver that helps you find solution for quadratic equations using the quadratic formula.

Solution First we notice that the -7 term must be replaced if we are to have a perfect square trinomial, so we will rewrite the equation, leaving a blank for the needed number. From your experience in factoring you already realize that not all polynomials are factorable. In this section we will derive and use a formula to find the solution of a quadratic equation. In a short moment, the calculator will show the roots. The quadratic formula is important because it allows us to solve quadratic equations, which are commonly found in many different disciplines. Step 6 Solve for x two values. How to Use the Quadratic Equation Calculator? When using imaginary numbers, however, you cannot graph a parabola on an x-y plane like usual. Welcome to MathPortal. Quadratic Equations with steps. By inputting the equation, users can find out its roots instantly. Step 1 : Divide the equation by the number in front of the square term. Simplify the radical.