Uiuc calc 2

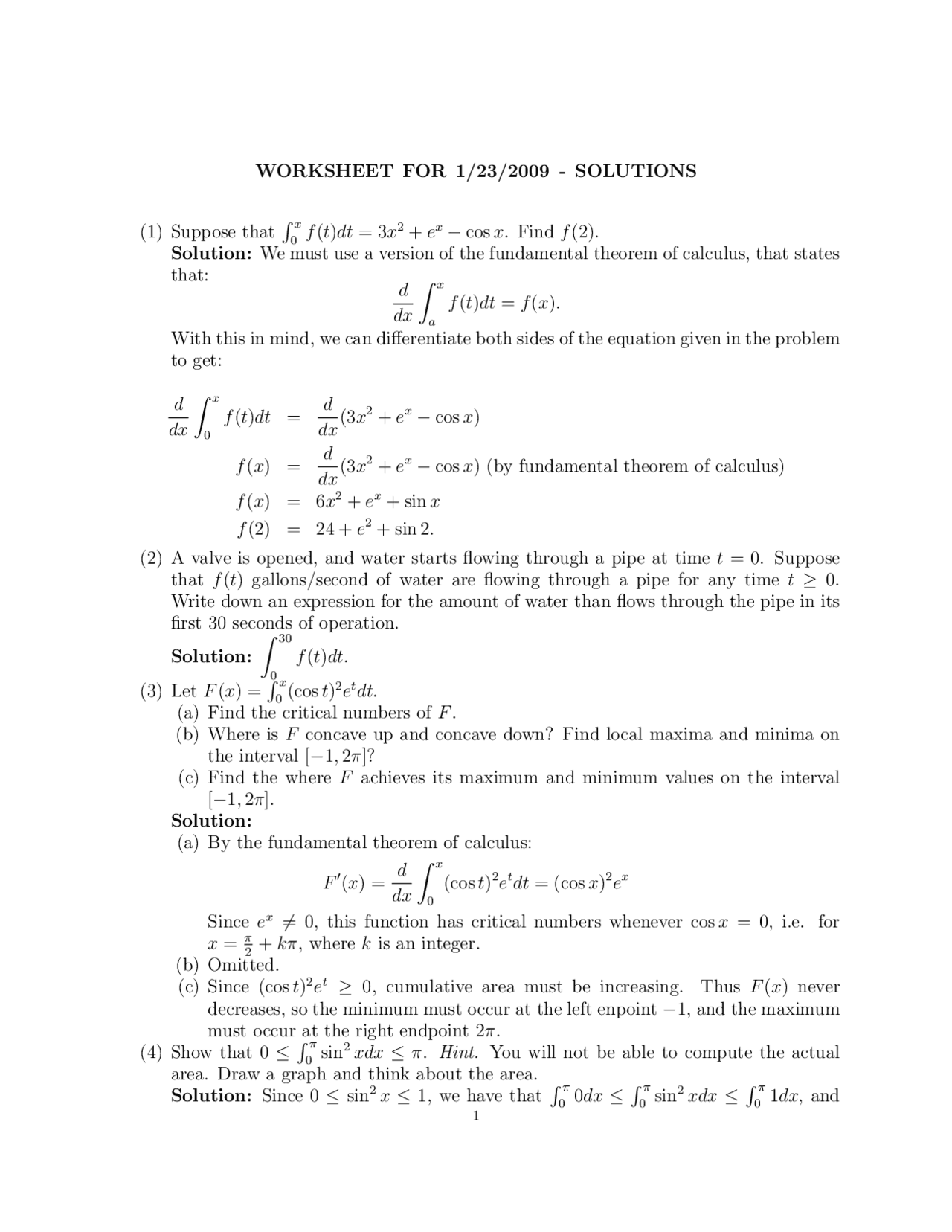
Analyses of the mathematical issues and methodology underlying elementary mathematics in grades K Topics include sets, arithmetic algorithms, uiuc calc 2, elementary number theory, rational and irrational numbers, measurement, and probability. There is an emphasis on problem solving. Priority registration will be given to students enrolled uiuc calc 2 teacher education programs leading to certification in elementary or childhood education.

Do you feel like you could go back today or in a month and get a 5 on the AP exam again? If not, it may not be a bad idea to retake calc 2. It is just a matter of how comfortable you are. In other words, if you ever want to do grad school, you need to be really good at calculus. Different people may have a different opinion, but most people I knew seem to think this way. It is harder here because the teachers are in general, worse than your high school teachers, especially in math.

Uiuc calc 2

Designed for students in majors that do not specifically require a mathematics course beyond the level of precalculus. Focus is on critical thinking and applications. All topics are covered from a contextual standpoint. Topics include proportional reasoning and modeling, functions, sets, consumer math, probability, and statistics. Other topics may be covered as time permits. Prerequisite: Three years of high school mathematics. Undergraduates only. Analyses of the mathematical issues and methodology underlying elementary mathematics in grades K Topics include sets, arithmetic algorithms, elementary number theory, rational and irrational numbers, measurement, and probability. There is an emphasis on problem solving. Priority registration will be given to students enrolled in teacher education programs leading to certification in elementary or childhood education. Rapid review of basic techniques of factoring, rational expressions, equations and inequalities; functions and graphs; exponential and logarithm functions; systems of equations; matrices and determinants; polynomials; and the binomial theorem. Studies degrees and radians, the trigonometric functions, identities and equations, inverse functions, oblique triangles and applications. Prerequisite: 1. Reviews trigonometric, rational, exponential, and logarithmic functions; provides a full treatment of limits, definition of derivative, and an introduction to finding area under a curve.

Midterms will be held during our usual class time.

Email : xinran4 illinois. Campuswire : This course will have a campuswire page where you can post and answer questions. I'll monitor campuswire during my office hour. Syllabus : Here is the Syllabus. We will cover Chapters 7, 8, 10 and 11 of the textbook, see also department syllabus.

The program prepares students for careers as actuaries and enterprise risk analysts through a curriculum that reflects the interdisciplinary nature of actuarial science. Students interested in teaching mathematics at the middle and high school level may complete a math major and the secondary education minor. Alternatively, they may complete a teaching program in another area and our minor in the teaching of mathematics, grades or grades The major and minor in statistics are available through the Department of Statistics. Students have the opportunity to gain research experience in the Illinois Mathematics Lab formerly known as the Illinois Geometry Lab where undergraduates work together with graduate students, postdocs, and faculty members on dedicated mathematics research projects. The IML also provides students with a wide array of opportunities to engage in campus and community outreach. Our students also participate in research year-round through the Math Honors Seminar, summer research experiences for undergraduates, and individually-arranged faculty reading courses. We have an active team of creative problem-solvers who prepare in the fall for the Putnam Exam, a nationwide mathematics competition for undergraduates, and keep in practice with the U of I Undergraduate Math Contest each spring.

Uiuc calc 2

Designed for students in majors that do not specifically require a mathematics course beyond the level of precalculus. Focus is on critical thinking and applications. All topics are covered from a contextual standpoint. Topics include proportional reasoning and modeling, functions, sets, consumer math, probability, and statistics. Other topics may be covered as time permits. Prerequisite: Three years of high school mathematics. Undergraduates only. Analyses of the mathematical issues and methodology underlying elementary mathematics in grades K Topics include sets, arithmetic algorithms, elementary number theory, rational and irrational numbers, measurement, and probability. There is an emphasis on problem solving.

Fiesta 2005 precio

Group actions with applications. Students will regularly write proofs emphasizing precise reasoning and clear exposition. Applications to configuration and phase spaces, Maxwell equations and relativity theory will be discussed. I said that if he wanted to, he could probably do it over the course of the full 10 weeks people regularly take 4 of them over the normal 16 week semesters. An introduction to the study of dynamical systems. Students will regularly write proofs emphasizing precise reasoning and clear exposition. Examination of the historical origins and genesis of the concepts of the calculus; includes mathematical developments from the ancient Greeks to the eighteenth century. May be repeated with approval. Basic knowledge of matrix theory will be assumed. I did pass Calculus II, but the regular version, and that was very hard too. Covers the local and global structure of symplectic manifolds, their submanifolds, the special automorphisms they support Hamiltonian flows , their natural boundaries contact manifolds , their special geometric features almost complex structures , and their symmetries. See Class Schedule for current topics. Just wondering whether anyone has any first hand knowledge. Overview of the major components of modern machine learning, including linear and logistic regression, Principal Component Analysis, Support Vector Machines, feature importance, casual inference, training, validation and testing.

.

Topics include the Real number system and field axioms, sequences and series, functions and math modeling with technology, Euclidean and non-Euclidean geometry, probability and statistics. Jordan-Holder theorem. Introductory course emphasizing techniques of linear algebra with applications to engineering; topics include matrix operations, determinants, linear equations, vector spaces, linear transformations, eigenvalues, and eigenvectors, inner products and norms, orthogonality, equilibrium, and linear dynamical systems. Prerequisite: Three years of high school mathematics, including two years of algebra and one year of geometry. Prerequisite: Consent of instructor. Students will regularly write proofs emphasizing precise reasoning and clear exposition. Students will learn how to implement linear algebra methods on a computer, making it possible to apply these techniques to large data sets. Full-time or part-time practice of math or actuarial science in an off-campus government, industrial, or research laboratory environment. Prerequisite: Consent of the department. A commonly recommended source is Paul's online notes Prof. Instead, they are thorough in teaching, collect homework on a regular basis, and usually give fair exams. There is an emphasis on problem solving. Topics and nature of assistance vary.